First, use the following formula to determine the time the bull takes to reach the target:
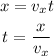
where,
x: horizontal distance to the target = 180m
vx: horizontal component of the velocity = 8.0*10^2 m/s
Replace the previous values of the parameters into the expression for t:
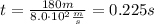
Next, use the previous time to calculate the vertical distance traveled by the bullet just before it hits the target, as follow:
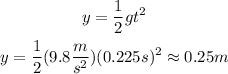
Hence, the bullet fals approximately 0.25m before it hits the target.