Answer:
The limit of the function exists
The value of the limit is 1/8
Explanations:
Given the limit of the function:
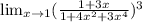
In order to get the limit of the function, we will substitute x = 1 into the function;
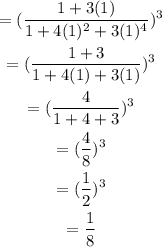
Since the limit of the function results in a finite value, hence the limit exists.
The value of the limit is 1/8