1 Answer

Shawnr · Answer 1 · 2023-09-01T01:35:05+0000

The volume of the solid object is 12 cubic units

Note that dilating an object with a scale factor of k will reduced or enlarge the measurement of it's side.

For the volume, we have three dimensions since its in the cubic form..

For example :

length x width x height (Three dimensions)

So to get the new volume :

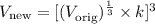
$V_{\text{new}}=\lbrack(V^{}_{\text{orig}})^{(1)/(3)}* k\rbrack^3$

The original volume must be raised to 1/3 to get one unit dimension..

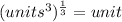
$(units^3)^{(1)/(3)}=unit$

Substitute the original volume and the values of k to the formula :

For k = 1/4

$V_(new)=(12^{(1)/(3)}*(1)/(4))^3_{}=(3)/(16)=0.188$

For k = 0.4

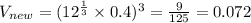
$V_(new)=(12^{(1)/(3)}*0.4)^3_{}=(9)/(125)=0.072$

For k = 1

Volume will still be the same since the scale factor is 1.

For k = 1.2

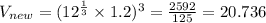
$V_(new)=(12^{(1)/(3)}*1.2)^3_{}=(2592)/(125)=20.736$

For k = 5/3

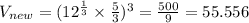
$V_(new)=(12^{(1)/(3)}*(5)/(3))^3_{}=(500)/(9)=55.556$

For k = 6.1

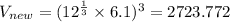
$V_(new)=(12^{(1)/(3)}*6.1)^3_{}=2723.772$

Note that all answers are in cubic units.

Please log in or register to add a comment.