Answer: The width is x + 4
Step-by-step explanation
• The area (,A,) is:

• The length (,l,) is:
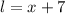
The formula for the area of a rectangle is:
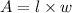
where w represents the width.
Then, to get the width we have to isolate it in the formula:
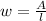
Replacing the expressions given:
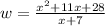
To simplify this expression we can factor the numerator by finding two numbers that when added equal 11, and when multiplied equal 28. For example, 4 and 7:
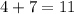
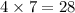
Then, the expression is:

Simplifying:

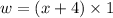
Then the width is x + 4.