Question

The points scored on a test for a sample of 46 students are summarized in the following table.Number of students Score for each student

Answer 1

Given a table for the points scored on a test for a sample of 46 students.

The mean of data is

`\bar{X}=\frac{\text{ sum of observations }}{\text{ number of observations}}`

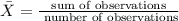
Here, the score of 9 students is given 90 each. So, the sum of scores of these 9 students is 810.

The score of 17 students is given 80 each. So, the sum of scores of these 17 students is 1360.

The score of 12 students is given 70 each. So, the sum of scores of these 12 students is 840.

The score of 8 students is given 60 each. So, the sum of scores of these 8 students is 480.

Now, the sum of scores of all 46 students is 3490.

So, the mean of the given data is calculated below:

`\begin{gathered} \bar{X}=(3490)/(46) \\ =75.9 \end{gathered}`

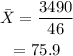
Thus, the mean score is 75.9.