Start writing the corresponding system of equations taking into account that each dime is equal to $0.1 and each quarter is equal to $0.25, let d be the number of dimes and q the number of quarters.
then,
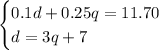
insert the second equation into the first one
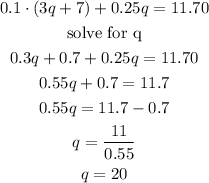
find the number of dimes using the number found and the second equation

prove the solution using the first equation
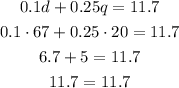
There are 67 dimes and 20 quarters.