Rachel is wrong, because she is claiming that
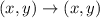
Is a reflection over the y-axis. because
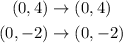
A reflection over the y-axis is actually represented as:
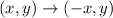
But because both of the points that Rachel chose have an x coordinate of 0, and -0 = 0 , Rachel thought that her reasoning was right, when it wasn't.
In fact, every point that is in the y-axis (x coordinate = 0) its mapped to itself when reflected over the y axis.
This way, the correct answer is:
C. Rachel's claims is incorrect since her examples are both in the y-axis