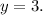Question

Circle A is located at (6, 5) and has a radius of 2 units. What is the equation of a line that is tangent to circle A from point C (1, 3)? y = −2.5x + 12.75 y = 6 y = 0.4x + 2.6 y = 3

Answer 1

Given:

The center of circle A is (6,5).

The radius of the circle is 2 units.

The point on the tangent is C(1,3).

Required:

We need to find the equation of the tangent.

Step-by-step explanation:

The tangent is a straight line which is of the form

`y-y_1=m(x-x_1)`

Where m is the slope of the tangent.

`\text{ Substitute }x_1=1\text{ and }y_1=3\text{ in the equation.}`

`y-3=m(x-1)`
`y=m(x-1)+3`

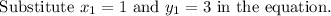
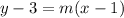
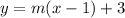
Consider the circle equation.

`(x-h)^2+(y-k)^2=r^2`

Substitute h =6, k=5, and r =2 in the formula.

`(x-6)^2+(y-5)^2=2^2`
`(x-6)^2+(y-5)^2=4`

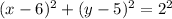
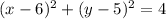
Substitute x=1 in the equation, and we get

`(4-6)^2+(y-5)^2=4`
`y-5=0`
`y=5`

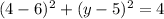
We get the point that lies in the circle is (4,5).

Use the points (4,5) and (6,5) to find the slope of the radius.

`m=(5-5)/(6-0)=0`

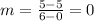
We know that the radius and tangent are perpendicular to each other.

The slope of the perpendicular lines is negative reciprocal.

The slope of teh tangent line is again zero.

`Subsitute\text{ m =0 in the equation }y=m(x-1)+3.`
`y=3`

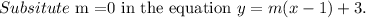
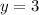
Final answer:

The equation of the tangent line from point C(1,3) is

`y=3.`