Question

Hello just wanted to make sure both parts are correct and if there’s anything I should add. Thanks for your help!

Answer 1

Given the functions:

`\begin{gathered} f(x)=x^4-4x^3-2x^2+12x+9 \\ \\ g(x)=√(x^2-2x-3) \\ \\ h(x)=(-x^2+1)/(x^2-2x-3) \end{gathered}`

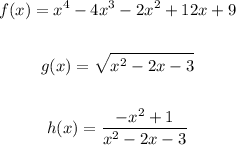
Let's answer the following questions.

• (a). Let's compare the domain and range of f(x) and g(x) using statements.

Domain and range of f(x):

Since f(x) is a polynomial function, the domain will be all real numbers:

Domain: (-∞, ∞)

The range will be:

Range: [0, ∞)

• Domain and range of g(x):

The domain of g(x) is:

Domain: (-∞, -1] ∪ [3, ∞)

The range of g(x) is:

[0, ∞)

Therefore, using complete statements, we have:

The domain and range of f(x) are D(-∞, ∞) and R [0, ∞), while the domain and range of g(x) are D(-∞, -1] ∪ [3, ∞) and R[0, ∞)

• (b). The breaks of the function h(x) is defined by the denominator of the rational function h(x)

Using the function, h(x), the zeros of the denominator are:

x = -3, 1

The zeros of f(x), are:

x = -3, 1

Therefore, the breaks in the domain of h(x) are at x = -3 and x = 1 which is equal and located at the same point as the zeros of the domain of f(x).

ANSWER:

• (a). The domain and range of f(x) are D(-∞, ∞) and R [0, ∞), while the domain and range of g(x) are D(-∞, -1] ∪ [3, ∞) and R[0,, ,∞).

• (b). The breaks in the domain of h(x) are at x = -3 and x = 1 which is equal and located at the same points as the zeros of the domain of f(x).