Question

Hey pls help me out with this & show your workFind the surface area of each solid. Round to the nearest tenth of necessary.

Answer 1

To calculate the surface area of a rectangular prism you have to calculate the area of each face of the prism and then add all areas.

The rectangular prism has 6 faces and the opposite sides are congruent, which means that there are three pairs of rectangular shapes:

1) Bottom and top faces:

The dimensions of these sides are determined by the width and length of the prism, you can determine their area using the formula:

`2A_1=2wl`

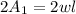
The width of the prism is w=4in and the length is l=12in, so the area of these faces can be determined as follows:

`\begin{gathered} 2A_1=2\cdot4\cdot12 \\ 2A_1=2\cdot48 \\ 2A_1=96in^2 \end{gathered}`

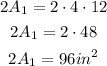
2) Front and back faces:

The dimensions of these faces are given by the height and length of the prism, you can calculate the area of both faces as follows:

`2A_2=2hl`

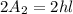
The height of the prism is h=4in and the length is l=12in, so the areas can be calculated as follows

`\begin{gathered} 2A_2=2\cdot4\cdot12 \\ 2A_2=2\cdot48 \\ 2A_2=96in^2 \end{gathered}`

3) Left and right faces:

The dimensions of these faces are determined by the width and the height of the prism, their area can be calculated as follows:

`2A_3=2wh`

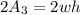
The width is w=4in and the height is h=4in, the areas can be calculated as follows:

`\begin{gathered} 2A_3=2\cdot4\cdot4 \\ 2A_3=2\cdot4^2 \\ 2A_3=2\cdot16 \\ 2A_3=32in^2 \end{gathered}`

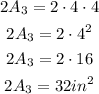
Once all areas are determined, you have to add them to determine the surface area (SA) of the prism:

`\begin{gathered} SA=2A_1+2A_2+2A_3 \\ SA=96+96+32 \\ SA=224in^2 \end{gathered}`