Question

Point charges q1 = 50 µC and q2 = −75 µC are placed 1 m apart. Determine the magnitude and direction of the force on a third charge q3 = 20 µC placed midway between q1 and q2

Answer 1

The force which acts on the third charge placed in between the two charges can be expressed as,

`\begin{gathered} F=(kq_1q_3)/(r^2)+\frac{kq_{2_{}}_{}q_3}{r^2} \\ =(kq_3)/(r^2)(q_1+q_2) \end{gathered}`

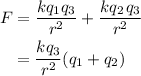
Here, F is the net force, k is the force constant, q1 is the first charge, q2 is the second charge, q3 is the second charge and r is the distance between first and third charge. Since the third charge is placed in midway of both the charges therefore,

`\begin{gathered} r=\frac{1\text{ m}}{2} \\ =0.5\text{ m} \end{gathered}`

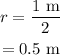
Plug in the known values in the expression,

`\begin{gathered} F=\frac{(9*10^9Nm^2C^(-2))(20\text{ }\mu C)(\frac{10^(-6)\text{ C}}{1\text{ }\mu C})}{(0.5m)^2}(50\text{ }\mu C-75\text{ }\mu C) \\ =(720*10^3\text{ N/C)(-}25\text{ }\mu C)(\frac{10^(-6)\text{ C}}{1\text{ }\mu C}) \\ =-18\text{ N} \end{gathered}`

Therefore, the net force which acts on the third charge is -18 N in which negative sign indicates that the direction of force is towards the negative charge of the system.