Question

Write an equation of the circle with a center at (5, -2) and passing through (4,0).

Answer 1

`(x-5)^2+(y+2)^2\text{ = 5}`

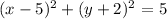
Step-by-step explanation

We want to find the equation of the circle that has center (5, -2) and passes through (4, 0).

To do this we use the formula:

`(x-h)^2+(y-k)^2=r^2`

where (h, k) = center of the circle = (5, -2)

r = radius of the circle

To find the radius of the circle, we use the formula for the distance between two points (center and radius):

`r\text{ = }\sqrt[]{(x_2-x_1)^2+(y_2_{}-y_1)^2}`

where (x1, y1) = (5, -2)

(x2, y2) = (4,0)

Therefore:

`\begin{gathered} r\text{ = }\sqrt[]{(4-5)^2+(0-(-2))^2} \\ r\text{ = }\sqrt[]{(-1)^2+2^2} \\ r\text{ = }\sqrt[]{1\text{ + 4}} \\ r\text{ = }\sqrt[]{5} \end{gathered}`

So, we have that:

`\begin{gathered} (x-5)^2+(y-(-2))^2\text{ = (}\sqrt[]{5})^2 \\ \Rightarrow\text{ }(x-5)^2+(y+2)^2\text{ = 5} \end{gathered}`

That is the equation of the circle.