Question

1. Consider the following diogrom that displays a mop of a set of circular running frocio in park connected by a sidewolk that goes from point on the circle that represents the outer trock to the center of all the circles, K (E K o is 100 meters long Ke of the length of X and XD s of the length of Ka Joe vits The pork every morning to run a lap around each of the three circular tracks He starts ung trom po going one lop ground the outer crcor Mock. Once joe completes Hotloo he run from pont A to point to complete another loo around the second culortrack and then runs from pont to contato complete re linalo around me accock once love third op beans Bom going to point and so there for less to costa Suppose that the stone un round eache to the ones of Delincunyod

Answer 1

In this case, we'll have to carry out several steps to find the solution.

Step 01:

Data

ED = 100 m

total distance run by Joe = ?

Step 02:

KE = 1/4 KA

ED = 100 m

KD = 3/4 KA

KD = KE + ED = 3/4 KA

KD = 1/4 KA + 100 = 3/4 KA

1/4 KA + 100 = 3/4 KA

`\begin{gathered} (1)/(4)KA\text{ - }(3)/(4)KA\text{ = - 100} \\ (-2)/(4)KA=-100 \\ KA\text{ =( 100)}\cdot((4)/(2)) \end{gathered}`

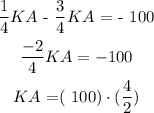
KA = 100*2 = 200 m

KE = 1/4 KA = 1/4 (200) = 50 m

KD = 3/4 KA = 3/4 (200) = 150 m

Step 03:

Circumference of circle

C = 2 π r

Total distance run by Joe:

distance 1:

C1 = 2 π r = 2*π* 50m = 314.159 m

C2 = 2 π r = 2*π* 150m = 942.478 m

C3 = 2 π r = 2*π* 200m = 1256.637 m

distance 2:

AD = KA - KD = 200 m - 150 m = 50m

DE = 100 m

distance 3:

KE = 50 m

total distance run by Joe = C1 + C2 + C3 + AD + DE + KE = 2713.274 m

The answer is:

The total distance run by Joe is 2713.274 m