Step-by-step explanation
Since we have the given sequence:
![\mathrm{An\: arithmetic\: sequence\: has\: a\: constant\: d}if\mathrm{ference\: }_{\text{ }}d_{\text{ }}\mathrm{\: and\: is\: defined\: by}\: a_n=a_1+\mleft(n-1\mright)d]()
Computing the differences of all the adjacent terms:
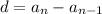

The difference is not constant, thus the sequence is not arithmetic.
b) Applying the same reasoning to the second sequence:
4.5, 9,18,36
Computing the difference of all the adjacent terms:
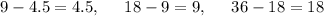
The difference is not constant.
Therefore, the sequence is not an arithmetic one.