Given:
The resistance of the electric blanket, R_b=14.1 Ω
The resistance of the tv, R_t=26.1 Ω
The resistance of the light bulb, R_l=34.5 Ω
The supply voltage, V=113 V
To find:
a. Equivalent resistance of the circuit.
b. The total current in the circuit.
c. The current through the electric blanket.
Step-by-step explanation:
a. The equivalent resistance of the resistors that are connected in parallel is given by,
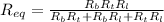
On substituting the known values,
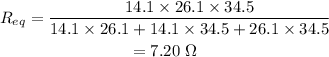
b.
From Ohm's law, the potential difference in the circuit is given by,
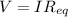
Where I is the total current in the circuit.
On substituting the known values,
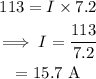
c.
As the electric blanket is connected in parallel, the potential difference across it is V.
Thus, from Ohm's law,
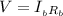
On substituting the known values,
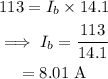
Final answer:
a. The equivalent resistance of the circuit is 7.20 Ω
b. The total current in the circuit is 15.7 A
c. The current through the electric blanket is 8.01 A