Let's first determine the zeros of the given polynomial:
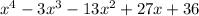
One of the factors is given, x - 4.
To summarize, the factors are:
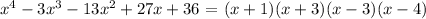
This polynomial is a positive even power (in particular, it's of degree four), so the graph will go up on both ends.
So the zeroes (that is, the x-intercepts (at y = 0)) are at:
x = -1, -3, 3, 4
If we plot a few other points on our T-chart, it will be no trouble to do the graph. Because the pairs of x-intercepts are so close, I'll go to the trouble of evaluating the polynomial at the halfway points between the zeroes; that is, at x = -2 and x = 3.5.
This polynomial is a fourth power, so it likely grows quickly. So I'll do one point below and another above the zeroes.
Substituting the given equation by a number of possible x - values.
x x^4-3x^3-13x^2+27x+36 = y
-4 168
-2 -30
0 36
3.5 -7.3125
5 96
Plotting the graph will be: