Question

give the rectangular form of the complex number represented in the graph.A. -5 -2iB. 5-2iC. 5+2iD. -5+2i

Answer 1

`z=a+bj`

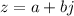
The rectangular form of a complex form is given in terms of 2 real numbers a and b in the form: z=a+jb

The polar form of the same number is given in terms of a magnitude r (or length) and argument q (or angle) in the form: z=r|_q

You can "see" a complex number on a drawing in this way:

`\begin{gathered} a=-5 \\ b=2 \\ a+bj \\ -5+2i \end{gathered}`

In this case the numbers a and b become the coordinates of a point representing the complex number in the special plane (Argand-Gauss) where on the x axis you plot the real part (the number a) and on the y axis the imaginary (the b number, associated with j).

Therefore the correct answer is -5 + 2i

Hence the answer is Option D