Given:
The function is,
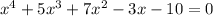
Use the synthetic division,
The rational roots are of the form,

As, the constant term is -10, its factors are
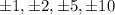
These are the possible values of p.
Also, the leading coefficient is 1. its factors are

These are the values of q.
From the above synthetic division, the actual rational roots are,

Answer: rational roots are -2, 1