Question

What is the equation of the following line? Be sure to scroll down first to seeall answer options.(0, 0)(-2,-3)A. y = 3xB. y = xc. y = 23 xD. yX

Answer 1

Ok, the equation of a line is given by two parameters:

`y=a\cdot x+b`

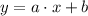
Where the parameters are a and b, the slope and the intercept. The intercept is the y-value where the graph of the line intercepts the y-axis while the slope describes how fast the y-value in the former expression increases or decreases. To find the slope you need at least two points that belong to the line. Assuming those points are (c,d) and (e,f) then the slope is given by:

`a=(f-d)/(e-c)`

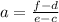
In this case we have points (0,0) and (-2,-3) so the slope is given by:

`(-3-0)/(-2-0)=(-3)/(-2)=(3)/(2)`

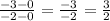
So the equation of the line is:

`y=(3)/(2)x+b`

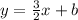
To find b we just need to pick one of the two points we have and replace x and y in the equation above with the coordinates of the point chosen. Let's use (-2,-3):

`\begin{gathered} y=-3 \\ x=-2 \\ -3=(3)/(2)\cdot(-2)+b \\ -3=-3+b \\ -3+3=0=b \end{gathered}`

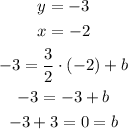
Then b=0 so the equation of the line is:

`y=(3)/(2)x`

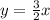
So the correct option is C.