Question

What is the molarity of a nitric acid solution if 49.90 mL of a 0.01 KOH solution is needed to neutralize 20.00 mL of the acid solution?

Answer 1

The question requires us to calculate the molarity (or molar concentration) of a HNO3 solution, given the volume and concentration of a KOH solution used to neutralize a given volume of the acid.

The following information was provided by the question:

molarity of KOH solution = M(KOH) = 0.01 M = 0.01 mol/L

volume of KOH solution = V(KOH) = 49.90 mL

volume of HNO3 solution = V(HNO3) = 20.00 mL

To answer this question, we need to take a look at the neutralization reaction between HNO3 and KOH:

HNO3 + KOH -> KNO3 + H2O

From the reaction, we have that 1 mol of HNO3 is necessary to neutralize 1 mol of KOH. With this information, we can write that, to achieve complete neutralization:

number of moles of HNO3 = number of moles of KOH

Since the molarity of a solution is given by the following equation:

`\text{molarity = }\frac{\text{number of moles (mol)}}{volume\text{ of solution (L)}}\to M=(n)/(V)`

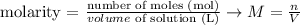
We can rearrange it to:

`n=M* V`

And we can also write the equality between the number of moles of HNO3 and KOH as:

`n_(HNO_3)=n_(KOH)\to M_(HNO_3)* V_(HNO_3)=M_(KOH)* V_(KOH)`

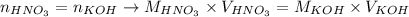
And, since the concentration of KOH was provided, as well as the volumes of HNO3 and KOH necessary to achieve neutralization, we can apply these values to calculate the molarity of HNO3 using the equation above:

`M_(HNO_3)* V_(HNO_3)=M_(KOH)* V_(KOH)\to M_(HNO_3)=(M_(KOH)* V_(KOH))/(V_(HNO_3))`
`M_(HNO_3)=\frac{(0.01\text{ mol/L)}*(49.90\text{ mL)}}{(20.00\text{ mL)}}=0.02495\text{ mol/L}`

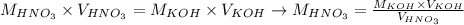
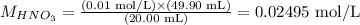
Therefore, the molarity of the nitric acid solution is equal to 0.02495 mol/L.