Conditional Probability : The probability of one event occurring with some relationship to one or more other events.
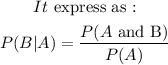
In the given question we have :
P(A) = .4, P(B) = .2 and P(A/B) = 0.6
a) P(A and B)
Simplify the general expression of conditional probability for P(A and B)
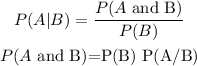
Substitute the value in the given expression :

P( A and B ) = 0.12
b) P(B/A)
Now again simplify the general expression for P(B/A)
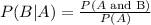
Substitute the value and simplify :
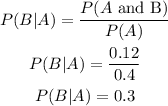
P(B|A) = 0.3
Answer : P( A and B ) = 0.12
P(B|A) = 0.3