Question

The numbered disks shown are placed in a boxand one disk is selected at random. Find theprobability of selecting a 6, given that a blue diskis selected.Find the probability of selecting a 6, given that a blue disk is selected.(Type an integer or a simplified fraction.)

Answer 1

The probability of selecting a 6, given that a blue disk is selected is given by the following formula:

`P(B|A)=P(A\text{ n B\rparen/P\lparen A\rparen}`

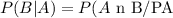
There are 4 blue in : 1, 2 , 6, 8

P(A)= blue disk selected:

P(B)= 6 is selected

`P(A)=(4)/(8)=(1)/(2).`

`P(A\text{ }n\text{ B})=P(A)*P(B)=(1)/(2)*(1)/(4)=(1)/(8)`

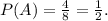
Where P(B) is the probablity to select the number 6 in four blue disks.

Substituing:

`\begin{gathered} P(A|B)=((1)/(8))\text{ /\lparen1/2\rparen} \\ \\ P(A|B)=(1)/(4) \end{gathered}`

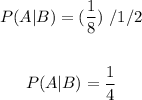
Answer: The probablity to select a 6, given that a blue disk was selected is: 1/4.