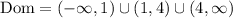We have:
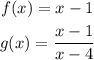
And we must find the quotient f/g. So let's take f and divide it by g:
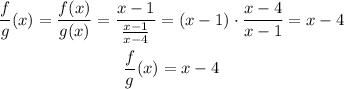
So the result is x-4. In order to calculate its domain we must take into acount that it was made from dividing f by g so this isn't a simple linear function with a domain from negative infinite to positive infinite. Any number that isn't part of the domain of g or that makes g=0 is a number that doesn't belong to the domain of f/g either. Values that aren't part of the domain of g are those who make its divider equal to 0 so we have:
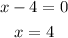
The values that make g=0 are those which make its dividend equal to 0:
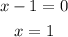
All these calculation mean that the values 1 and 4 are not part of f/g domain. Then the domain can be written as: