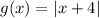The functions f(x) and g(x) are shown on the graph. f(x) = x|What is g(x) = ?A. g(x) = |X| - 4 B. g(x) = |x - 4|C. g(x) = |X+4| D. g(x) = |xi + 4

Question

asked Sep 5, 2023 135k views

1 Answer

Laurent Mazuel · Answer 1 · 2023-09-10T14:38:37+0000

Answer:

the function g(x) is;

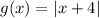
Step-by-step explanation:

Given the function f(x) to be;

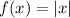
As shown on the graph the graph of f(x) and g(x) are plotted on the same graph.

f(x) was translated 4 units to the left to get g(x).

So, we can derive the function of g(x) as;

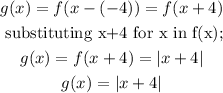
$\begin{gathered} g(x)=f(x-(-4))=f(x+4) \\ \text{ substituting x+4 for x in f(x);} \\ g(x)=f(x+4)=|x+4| \\ g(x)=|x+4| \end{gathered}$

Therefore, the function g(x) is;