Question

the ages (in years) of the 5 employees at a particular computer store are the following 35, 32, 37, 37, 34assuming that these ages constitute an entire population, find the standard devation of the population. round your answer to two decimal places.

Answer 1

The standard deviation is 1.90

The first thing we would do here is to find the mean of the given set

The mean can simply be calculated by adding all the numbers and dividing these numbers by their count

Mathematically, we have this as follows;

`(35+32+37+37+34)/(5)\text{ = }(175)/(5)\text{ = 35}`

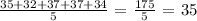
What we have to do next is to subtract the mean from each of the numbers

This would be;

35-35 = 0

32-35= -3

37-35 = 2

34-35 = -1

0 , -3 , 2 , 2 and -1

square each of these

0, 9 , 4 , 4 ,1

Sum of these is 18

Now, we use the standard deviation formula;

As we can see , the summation of all the numbers after subtracting the mean is 18

So, we have;

`\begin{gathered} \sigma\text{ = }\sqrt[]{(18)/(5)} \\ \sigma\text{ = }\sqrt[]{3.6} \\ \sigma\text{ = }1.90 \end{gathered}`