Question

The area of a great circle in a sphere is 364 square feet.Note: Find the exact answer for this question. Type the coefficient of pi in the boxes below. For example, if your answer was 10n, you wouldtype 10 in the box.The surface area of the sphere isn square feetThe volume of the sphere isn cubic feet.Blank 1:Blank 2:

Answer 1

Step 1 :

In this question, we have that:

The area of the great circle in a sphere is 36 pi square feet.

We are asked to find The surface area of the sphere and

The volume of the sphere.

Step 2:

We have that:

`\begin{gathered} Area\text{ of the circle = 36}\pi \\ \pi r^2\text{ = 36 }\pi\text{ } \\ \text{Divide both sides by }\pi\text{ , we have that:} \\ r^2\text{ = 36} \\ \text{square - root both sides, we have that:} \\ \text{r = 6} \end{gathered}`

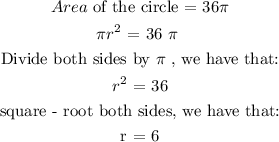
Step 3:

Next, we solve for the surface area of the sphere:

`\begin{gathered} \text{Surface Area of the Square= 4 x }\pi Xr^2 \\ \text{Now, we have that r = 6, then we have that:} \\ \text{Surface Area of the square = 4 X}\pi X6^2 \\ =\text{ 4 X }\pi\text{ X 36 } \\ =\text{ 144 }\pi\text{ square f}eet \end{gathered}`

The surface area of the sphere is :

`144\text{ }\pi\text{ square f}eet.`

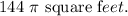
Step 4 :

Now, we have that:

`\begin{gathered} \text{Volume of the sphere = }(4)/(3)\text{ X }\pi Xr^3 \\ =\text{ }(4)/(3)\text{ X }\pi X6^3 \\ =\text{ }(4)/(3)\text{ X }\pi\text{ X 6 X 6 X 6} \\ =\text{ }(4)/(3)\text{ X }\pi\text{ X 216} \\ =\text{ 288 }\pi\text{ cubic f}eet \end{gathered}`

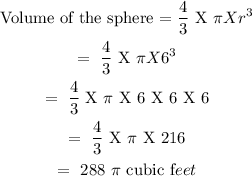
The volume of the sphere is:

`288\text{ }\pi\text{ cubic fe}et`