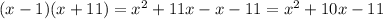Question

Enter the equation of a parabola that opens up with an axis of symmetry at x = -5 and one zero at 1.

Answer 1

The equation of the parabola is;

`x^2+10x-11`

The general equation of a parabola is;

`y=ax^2\text{ + bx + c}`

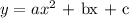
We have the axis of symmetry dividing the parabola into 2 equal halves

So, the distance from the axis of symmetry point to both roots are the same

So, the distance between - 5 and 1 is 1-(-5) = 6

This is the distance to the left

So, we need the distance to the right and that would be taking off 6 units from -5; so we have it as -11

So the equation is;

`(x-1)(x+11)=x^2+11x-x-11=x^2+10x-11`