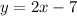Answer:
Given that,
To find an equation of the line that passes through the points (6, 5) and (7,7).
Step-by-step explanation:
we know that,
The equation of the line that passes through the points (x1,y1) and (x2,y2) is of the form,

x1=6 , y1=5
x2=7, y2=7
Substitute the values we get,
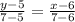
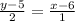
Solving this we get,
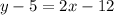
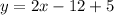
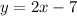
The required equation of the line is,