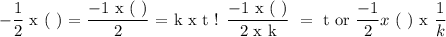2 N2O => 2N2 (g) + O2 (g)
We have an order to respect N2O, so:
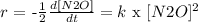
It says the reaction was carried out at 900K, so k doesn't change.
k depends on the temperature. That is important when we want to integrate.
Units:
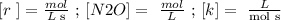
Let's separate variables and then integrate.

-1/2 and k goes out of the integral because they don't change.
We integrate and then we replace the values from the top and the bottom of the integrals
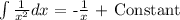
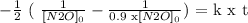
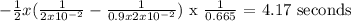
t = 4.17 s