We will have the following:
*First: We know that arithmetic sequences follow:
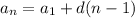
*Second: From the information given we will have:
a100:
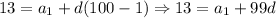
a200:

Then we will find the common difference:
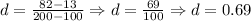
So, the common difference is 0.69.
*Third: We determine the first term:
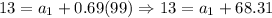
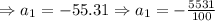
So, the first term is -55.31.