Question

Find the dimensions of the pen that will minimize the cost

Answer 1

First, we need to find the expression for the total cost C(x) of the fencing. Then, the value of x that minimizes the cost will be such as

C'(x) = 0

The exterior fencing costs 27.20 dollars per yard. So, if x and y are the dimensions of the pen, as shown in the image, the cost, in dollars, of the exterior fencing is:

(2x + 2y) * 27.20 = 2(x + y) * 27.2 = 54.4x + 54.4y

Notice that we can write y in terms of x because the total area inside the pen must be 1836 square yards. So:

x * y = 1836

y = 1836/x

Therefore, the exterior fencing will cost:

54.4x + 54.4 * 1836/x

Now, the cost of interior fencing is 16 dollars per yard. So, since there will be 2 interior fences, each one measuring x, the cost, in dollars, of interior fencing will be:

2x * 16 = 32x

So, the total cost will be:

C(x) = 32x + 54.4x + 54.4 * 1836/x

Then, the value of x that minimizes the cost is given by:

`\begin{gathered} C^(\prime)\mleft(x\mright)=0 \\ \\ 32+54.4+54.4\cdot1836\cdot(-(1)/(x^2))=0 \\ \\ 86.4=(99878.4)/(x^2) \\ \\ x^2=(99878.4)/(86.4) \\ \\ x^2=1156 \\ \\ x=\sqrt[]{1156} \\ \\ x=34 \end{gathered}`

And y that minimizes the cost is given by:

`\begin{gathered} y=(1836)/(x) \\ \\ y=(1836)/(34) \\ \\ y=54 \end{gathered}`

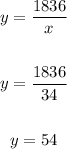
Therefore, the answer is:

x = 34 yards y = 54 yards