Question

A string is stretched from end to end oscillating at a frequency of 120 Hz. If the speed of sound on thestring is 80.9m/s, where should I put my finger (node) to create standing waves for the...1st Harmonic?2nd Harmonic?3rd Harmonic?Justify your answer.

Answer 1

We will have the following:

First, we determine the wave length, that is:

`\begin{gathered} \lambda=(v)/(f) \\ \\ \Rightarrow\lambda=(80.9m/s)/(120Hz)\Rightarrow\lambda=(809)/(1200)m \\ \Rightarrow\lambda=0.6741666666...m\Rightarrow\lambda\approx0.67m \end{gathered}`

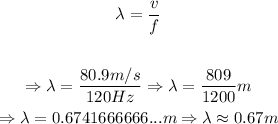
So, the wave length is approximately 0.67 meters.

Now, we determine the 1st, 2nd and 3rd harmonics, that is:

*1st harmonic:

`\begin{gathered} L=(1)/(2)\lambda\Rightarrow L\approx(1)/(2)(0.67m) \\ \\ \Rightarrow L\approx0.335m \end{gathered}`

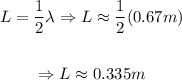
So, the first harmonic is at approximately 0.335 m.

*2nd harmonic:

`L=(2)/(2)\lambda\Rightarrow L\approx0.67m`

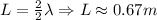
So, the second harmonic is located at approximately 0.67m.

*3rd harmonic:

`\begin{gathered} L=(3)/(2)\lambda\Rightarrow L\approx(3)/(2)(0.67m) \\ \Rightarrow L\approx1.005m \end{gathered}`

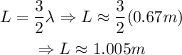
So, the third harmonic is located at approximately 1.005 m.