Given:
Minor axis length is 8 units.
Focci of elipse : (-7,4) and (7,4).
Required:
To find the equation of the elipse.
Step-by-step explanation:
The general equation of elipse is
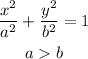
It is given that minor axis length is 8 units.
Therefore the value of b becomes 4.
Now we have to find the value of a.
And given that the focci points are (-7,4) and (7,4).
Here consider
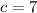
Now,
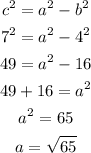
Therefore, the equation of the elipse becomes
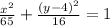
Final Answer:
