The line given is written in slope-intercept form as;
y=2/3x + 1
The slope is 2/3 and the y-intercept is 1. For a parallel line with the same slope (parallel lines on a coordinate grid have the same slope), we shall take the y-intercept at another point.
The line expressed as;

has the slope m as 2/3, and if it passes through the points (-3,1), then;
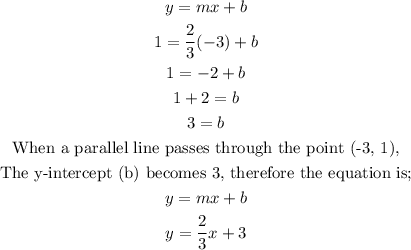
A parallel line that passes through the point (-3, 1) is given by the equation;
y = 2/3x + 3