Question

State theamplitude, period, vertical shift and phase shift for each. help idenity and create peace amongst the war field in my mind.

Answer 1

The general form of the cosine equation is

`y=AcosB(x-C)+D`

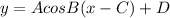
A is the amplitude

B is used to dine the period as period = 2pi/B

C is the phase shift

D is the vertical shift

Since the given equation is

`y=(1)/(2)cos(3x-180)-5`

Take from the bracket 3 as a common factor

`y=(1)/(2)cos3(x-60)-5`

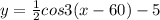
Compare it with the general form

`\begin{gathered} A=(1)/(2) \\ \\ B=3 \\ \\ C=60^(\circ)=(60)/(180)\pi=(1)/(3)\pi \\ \\ D=-5 \end{gathered}`

The amplitude is 1/2

The period = 2pi/3 = (2/3)pi ======== 120 degrees

The phase shift is (1/3)pi =========== 60 degrees

The vertical shift is -5