Answer:
• The amount invested at 6% is $1,150.
,
• The amount invested at 12% is $1,750.
Explanation:
Let the amount invested at 6% = x
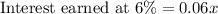
Kelvin has $600 more in the 12% account, therefore:
The amount invested at 12% = $(x+600).
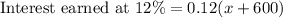
The total interest is $279.

We solve the equation for x.

The amount invested at 6% is $1,150.
The amount invested at 12% is:
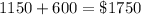
The amount invested at 12% is $1,750.