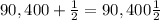ANSWER
90,400.5
Step-by-step explanation
To add these factors, first, we have to solve the multiplications in the parenthesis,
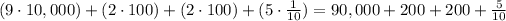
Note that the last term is a fraction, so first, we have to simplify it. Both numerator and denominator are divisible by 5, thus,
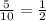
Add the whole numbers first,

Then we can either add the fraction so the answer is another fraction with a rather large numerator, or we can add the fraction as a decimal,
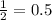
And the result is,
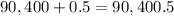
If we add the fraction and express the answer as an improper fraction we have,
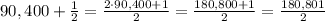
Or we can also write it as a mixed number,