Question

At a high school football game, admission is $5 for students and $10 for non-students. The school made $4,000 from admissions at the first game,which 500 people attended. How many students and non-students were at the game? Earlier you wrote the system of equations that models thissituation. In this activity, you will solve this system of equations.S+ n = 5005s + 10 = 4,000Part ALooking at the two equations, which method should you use to solve this system of equations?

Answer 1

`\begin{gathered} s+n=500 \\ 5s+10n=4000 \end{gathered}`

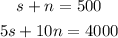
To solve the system of equation use substitution method:

1. Solve s in the first equation:

`s=500-n`

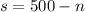
2. Substitute in the second equation the s for the value you get in step 1:

`5(500-n)+10n=4000`

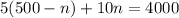
3. Solve n:

`\begin{gathered} 2500-5n+10n=4000 \\ -5n+10n=4000-2500 \\ 5n=1500 \\ n=(1500)/(5) \\ \\ n=300 \end{gathered}`

4. Use the value of n to solve s:

`\begin{gathered} s=500-n \\ s=500-300 \\ s=200 \end{gathered}`

Then, in the game were 200 students and 300 non-students