Question

What is the area of the pentagon with a radius of 7mm?

Answer 1

Given:

Radius of the pentagon = 7 mm

Let'd find the area of the pentagon.

A pentagon is a polygon with 5 sides.

To find the area of a pentagon, let's first find the length of each side of the pentagon.

We have a sketch below:

Apply the trigonometric ratio formula for tangent:

`tan\theta=\frac{opposite\text{ }}{adjacent}`

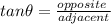
Where:

θ = 36 degrees

opposite side = x

Adjacent side = 7 mm

Let's solve for x:

`\begin{gathered} tan36=(x)/(7) \\ \\ x=7tan36 \\ \\ x=5.09\text{ mm} \end{gathered}`

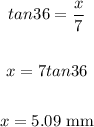
Therefore, the length of each side will be:

s = x + x = 5.09 + 5.09 = 10.18 mm

To find the area, apply the formula:

`A=(n*s*a)/(2)`

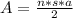
Where:

A is the area

n is the number of sides = 5

s is the length of each side = 10.18 mm

a is the radius = 7 mm

Thus, we have:

`\begin{gathered} A=(5*10.18*7)/(2) \\ \\ A=(356.006)/(2) \\ \\ A=178\text{ mm}^2 \end{gathered}`

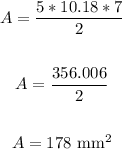
Therefore, the area of the pentagon is mm².

• ANSWER:

mm².