Question

In the figure, the area of the shaded region is 260cm^2what is the value of x?

Answer 1

x=8

Step-by-step explanation

Step 1

to find the area of the shaded region, we have to subtract the area of the small circle(yellow) from the area of the bigger circle( purple)

so

the area of a circle is given by

`\begin{gathered} A_(circle)=\pi r^2 \\ \text{where r is the radius} \end{gathered}`

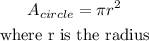
hence, replacing

`\begin{gathered} A_(region)=A_{big\text{ circle}}-A_{small\text{ circle}} \\ replace \\ 260\pi=\pi(18)^2-\pi(x^2) \\ 260\pi=\pi\cdot324-\pi(x^2) \\ 260\pi=\pi(324-x^2) \\ \text{divide both sides by pi} \\ 260=324-x^2 \\ \text{ssubtrac 324 in both sides} \\ 260-324=324-x^2-324 \\ -64=-x^2 \\ 64=x^2 \\ \text{square root in both sides} \\ \sqrt[]{64}=\sqrt[]{x^2} \\ x=\pm8 \\ we\text{ will take only the positive answer because we are looking for a distance} \\ x=8 \end{gathered}`

so, the answer is

x=8