We are given the following information
Before collision:
Mass of the 1st object = 18 kg
Initial speed of the 1st object = 23 m/s
Mass of the 2nd object = 17 kg
Initial speed of the 2nd object = 16 m/s
After collision:
Final speed of the 1st object = 3 m/s
Final speed of the 2nd object = ?
Recall from the law of conservation of momentum, the total momentum before the collision and after the collision must be equal.
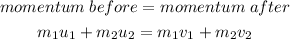
Let us substitute the given values and solve for the final speed of the 2nd object (v2).
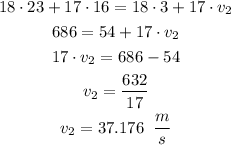
Therefore, the velocity of the 17 kg object after the collision is 37.176 m/s
Option D is the correct answer.