A committee must be formed with 4 teachers and 4 students. If there are 6 teachers to choose from and 12 students, how many different ways could the committee be made?
step 1
Find out the combination 4C6 (teachers)
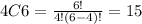
step 2
Find out the combination 4C12 (students)

step 3
Multiply 15 by 495
15*495=7,425 ways
therefore
the answer is 7,425 ways