We have the function:
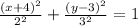
We can identify the nature of the shape by looking at it, from example, we know that the shape has a width of 4 (Given by the 2 at the power of 2) and has a lenght of 6 units (given by the 3 at the power of 2) [I'm not solving the powers just using the bases] and by the way it was written, we know the shape is centered around (-4, 3) and by the "+" between expressions and the relative positions of x and y in the function, we can deduce it belongs to an elipse.
Here is the graph of the function that shows that our assuptiom of an elipse is true [The negative x-axis section is represented from center to right and not as usually represented from left to center, this is a note so you won't get confused.]