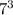Question

Write the quotient in repeated multiplication form. Then write the quotient as a power 7⁹---7⁶The quotient as repeated multiplication is The quotient as a power is

Answer 1

Given the indicinal expression;

`(7^9)/(7^6)`

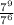
According to law of indices, when the base of the values are the same and they are dividing each other, we will subtract the power as shown;

`\text{Generally, }(a^m)/(a^n)=a^(m-n)`

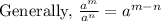
Simplifying the question now;

`\begin{gathered} (7^9)/(7^6)=7^(9-6) \\ (7^9)/(7^6)=7^3 \\ \end{gathered}`

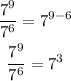
The quotient as repeated multiplication will be;

`7^3\text{ = 7}*7*7`

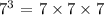
The quotient expresses as a power is;

`7^3`