Question

Find the area of the shaded areas. Round to the hundredths place.

Answer 1

From the diagram provided, the line segment WZ represents the radius of the circle. That means,

R = 5.3

Also, note that

`WZ=XZ=5.3`

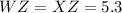
Next, observe that the angle formed by arc VW at VZW lies on a straight line with angle 108. Therefore,

`\begin{gathered} \angle VZW+108=180\text{ (angles on a straight line equals 180)} \\ \angle VZW=180-108 \\ \angle VZW=72 \end{gathered}`

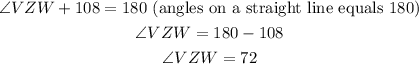
Also, arc VW equals arc XY, since both arcs are subtended by the same angle. Angle VZW and angle XZY are vertically opposite angles. Therefore we now have;

`\begin{gathered} \text{Area of a sector=}(\theta)/(360)*\pi* r^2 \\ \text{Area of a sector=}(72)/(360)*3.14*5.3^2 \\ \text{Area of a sector=}(1)/(5)*3.14*28.09 \\ \text{Area of a sector=}0.2*3.14*28.09 \\ \text{Area of a sector=17.64052} \end{gathered}`

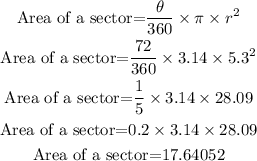
Note that there are two similar shaded sectors. Therefore the area of the shaded sectors equals;

Area = 17.64052 x 2

Area = 35.28104

Area = 35.28 km squared (rounded to the nearest hundredth)