The domain of an inequality is the set of all values that can be inputted into the function. That is, the set of all values that define the function.
The range of a function is the set of all values that are a function can take.
To indicate the domain of a function, we write:
![\left\lbrace x\right|aThis means that <strong>x takes all the values that are found between a and b.</strong><p>We can also write:</p>[tex]\left\lbrace x\right|a\leq x\leq b\}](https://img.qammunity.org/2023/formulas/mathematics/college/k8p6vufm47rtph8jwtx5zy97gb84actcva.png)
This indicates that x takes all values from a to b.
The range of a function is however written as:
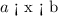
This indicates that the value of x is found to be between a and b.
It can also be written as:
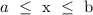
This indicates that the value of x is found to be ranging from a to b.
NOTE: Other inquality symbols can also be used