Question

A political candidate has asked you to conduct a poll to determine what percentage of people support her. If the candidate only wants a 10% margin of error at a 95% confidence level, what size of sample is needed? Give your answer in whole people.

Answer 1

Given:

margin of error = 10% or 0.10 in decimal form

confidence level = 95%

Find: sample size

Solution:

To determine the sample size, we can use Cochran's formula.

`n=(Z^2pq)/(e^2)`

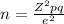
where:

Z is the critical value of the given confidence level

p = estimated proportion of the population having the attribute in question (ex. supports the candidate)

q = 1 - p

e = margin of error

Based on the given information, our confidence level is 95%. The z-value at this confidence level is 1.96. Hence, z = 1.96.

For p and q, since none is stated in the question, we will assume p = 0.5 and q = 0.5 or 50% each.

Let's plug into the formula above the values of Z, p, q, and e.

`n=(1.96^2(0.5)(0.5))/(0.10^2)`
`n=(3.8416*0.25)/(0.01)`
`n=(0.9604)/(0.01)\Rightarrow n=96.04\approx97`

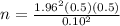
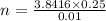
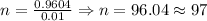
Any excess decimal will be considered as 1 person. So, 96 + 1 = 97.

Therefore, we need 97 people as our sample size.