Question

Find the perimeter and area of the triangle at the right. Round to the nearest tenth. I'll upload a picture.

Answer 1

Step-by-step explanation

We need to apply the Pythagorean Theorem in order to get the left unkwnown side:

`Hypotenuse^2=Smaller\text{ leg}^2+Larger\text{ leg}^2`

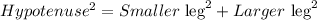
Plugging in the terms into the expression:

`14^2=9^2+larger\text{ leg\textasciicircum2}`

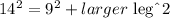
Computing the powers:

`196=81+larger\text{ leg}^2`

Subtracting -81 to both sides:

196 - 81 = larger leg^2

Applying the square root to both sides:

`√(115)=larger\text{ leg}`

Computing the root:

`larger\text{ leg=10.723}`

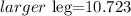
Now, with the obtained larger leg, we can compute the area, with the following equation:

`Area_(triangle)=(base*height)/(2)=(9*10.723)/(2)`

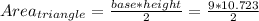
Multiplying numbers:

`Area_(triangle)=48.2in^2`

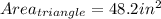
Now, we need to compute the perimeter by adding all the legs, as shown as follows:

`Perimeter=9in+14in+10.72in=33.72in`

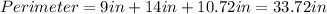
The solutions are the following:

Area= 48.2 in^2

Perimeter= 33.7 in