Given:
Principal, P= $1000
Interest rate, r = 0.8% = 0.008
Number of years, n = 25 years
To find the amount of money after 25 years, use the compound interest formula below.
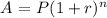
Thus, we have:
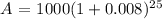

Therefore, the amount of money in the account after 25 years is $1,220.43
ANSWER:
$1,220.43