What we need here to answer this problem is to see the translation with respect to the angle C to the angle A.
The adjacent leg/hypotenuse of angle A is defined in the triangle as
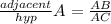
And base on angle C, this is the same as the opposite/hypotenuse of angle C wherein
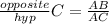
Therefore, the adjacent leg/hypotenuse of angle A is equal to 0.26.
The opposite/hypotenuse of angle A based on the figure is
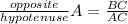
And base on angle C, this is the same as the adjacent/hypotenuse of angle C wherein
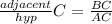
Therefore, the opposite/hypotenuse of angle A is equal to 0.97.
The opposite/adjacent of the angle A can be determined by just dividing the opposite/hypotenuse by the adjacent/hypotenuse. We have
![undefined]()